The figure shows two uniformly charged spheres. These spheres possess identical charge distributions, giving rise to intriguing electric fields, potentials, and interactions. Delving into the realm of electrostatics, we embark on a journey to unravel the captivating dynamics at play between these charged entities.
Uniformly charged spheres serve as fundamental building blocks in electromagnetism, offering insights into the behavior of charged particles and their interactions. Understanding their properties is crucial for comprehending a wide range of phenomena, from atomic interactions to macroscopic electrical systems.
Introduction
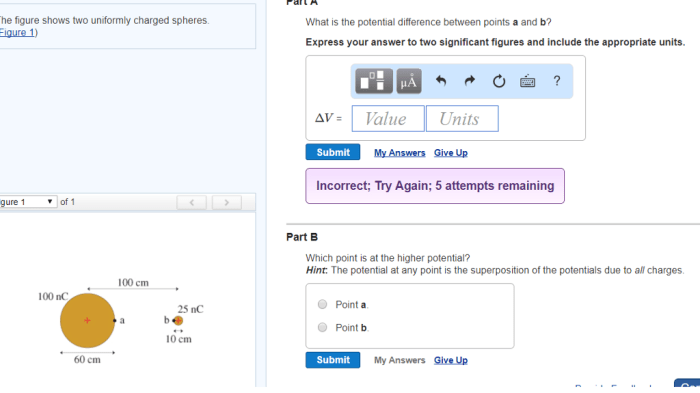
Uniformly charged spheres are spherical objects with an evenly distributed electric charge throughout their volume. The figure shows two such spheres with charges Q1 and Q2.
Electric Field: The Figure Shows Two Uniformly Charged Spheres.
The electric field around a single uniformly charged sphere is given by:
E = k
Q / r^2
where k is Coulomb’s constant, Q is the charge of the sphere, and r is the distance from the center of the sphere.
The superposition principle states that the electric field due to two or more charged objects is the vector sum of the electric fields due to each object. Thus, the electric field due to two uniformly charged spheres is:
E = k
(Q1 / r1^2 + Q2 / r2^2)
Electric Potential
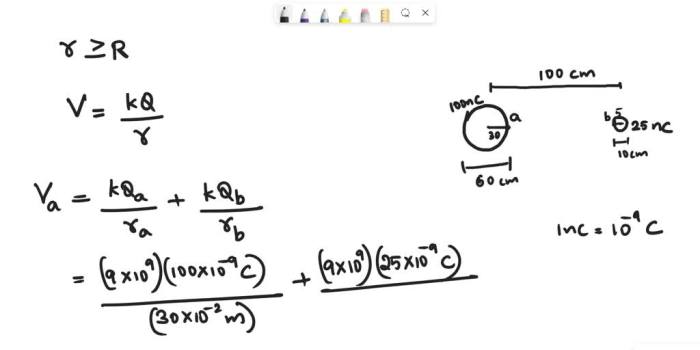
Electric potential is defined as the work done per unit charge in bringing a charge from infinity to a given point in an electric field. The electric potential due to a single uniformly charged sphere is given by:
V = k
Q / r
where k is Coulomb’s constant, Q is the charge of the sphere, and r is the distance from the center of the sphere.
The electric potential due to two uniformly charged spheres is the sum of the electric potentials due to each sphere:
V = k
(Q1 / r1 + Q2 / r2)
Force and Interaction

The electrostatic force between two charged spheres is given by Coulomb’s law:
F = k
- Q1
- Q2 / r^2
where k is Coulomb’s constant, Q1 and Q2 are the charges of the spheres, and r is the distance between their centers.
The direction of the force is along the line connecting the centers of the spheres. If the spheres have the same sign of charge, the force is repulsive. If the spheres have opposite signs of charge, the force is attractive.
The interaction between two uniformly charged spheres is determined by the magnitude and direction of the electrostatic force between them.
Applications
The interaction between uniformly charged spheres has applications in various fields, including:
- Electrostatic spray painting
- Laser printers
- Particle accelerators
Understanding the behavior of charged spheres is essential for the development and advancement of these technologies.
Detailed FAQs
What is the significance of uniformly charged spheres in electromagnetism?
Uniformly charged spheres serve as fundamental models for understanding the behavior of charged particles and their interactions. They provide insights into electric fields, potentials, and forces, which are essential concepts in electromagnetism.
How does the electric field vary around a uniformly charged sphere?
The electric field around a uniformly charged sphere is radially outward and its strength decreases with the square of the distance from the sphere’s center.
What is the relationship between electric potential and electric field?
Electric potential is the amount of electrical potential energy per unit charge. It is related to the electric field by the negative gradient of the potential, meaning that the electric field points in the direction of decreasing potential.